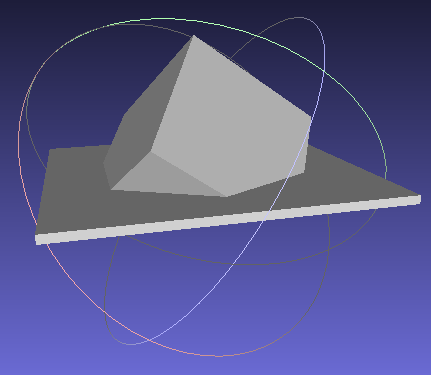
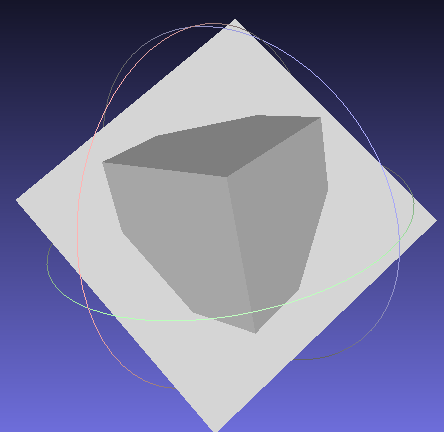
Half a Cube
In lattice QCD you have a qubic lattice. It has certain symmetries:
- Rotation around an axis perpendicular to a face. This goes with 90, 180 and 270 degrees.
- Rotation around an axis along a face diagonal. This goes with 180 degrees.
- Rotation around an axis along the volume diagonal. This goes with 120 and 240 degrees.
- There is also the inversion symmetry.
Taking all of them together you will get 48 elements, the octahedral symmetry group.
The question then was how the symmetries break down when we make a volume diagonal a special direction. In other words: What symmetries does a cube have when it is cut perpendicular to a volume diagonal? We know from group theory that it only has the rotations with 120 degrees left, but can one see that visually?
We tried to construct this on the blackboard and quickly failed to properly construct it. Luckily there is the programmer friendly OpenSCAD software with which I was able to construct exactly what we have in mind:
This makes it easy to see that the residual symmetry indeed are just the 120 degree rotations.